Staten Island (New York, USA) has grown to be a waste repository of trash over the last few centuries. The following excerpt appeared which astonished me in a recent article from the website 'Nautilus' titled "Reinventing Staten Island":
After World War II, the bursting city of New York found itself with a trash problem. In 1948, the city started officially dumping its trash into the marshes and waters of Fresh Kills. What became America’s first landfill was meant to be temporary, but it stuck. By 1955, it was the biggest landfill in the world—indeed, at 2,700 acres, it was the biggest human-made structure in the world. By 1991, the landfill contained 150 million tons of tightly packed garbage in more volume than could fill the Great Wall of China. Fresh Kills was wetland no more.
Specifically, the last sentence stood out among others. Which spurred the following questions in my mind:
1) How did a region manage to let 150 million tons of garbage collect in a single landfill?
2) What is the volume of the Great Wall of China?
3) How does the volume of the Great Wall of China compare to the stated value of 150 million tons?
The answer to the first question is complex and beyond my reach for an answer. I would defer the question to the politicians of the great state of New York. As for the next two questions, the overall interest which arose in my mind was to compare the stated value in the article to the actual (or approximate) volume of the Great Wall of China.
Basically, if I calculated a volume using approximate methods, how would that number (pounds of trash) compare to the value of 150 million tons? I will show below how to calculate the volume of the Great Wall of China and compare the answer to the stated value above.
How Big Is The Great Wall Of China?
When a reader encounters an enormous number such as "150 million tons" -- naturally the enormity will be difficult to comprehend. Which is why the process of 'dimensional analysis' is useful to cast an incomprehensible number into at least a semi-comprehensible number. The metric which the author chose in the excerpt above was the Great Wall of China shown below:
Source: History Channel
The dimensions of the Great Wall of China can be found by asking a search engine such as 'Google' the following: What are the dimensions of the great wall of china? The answer to which is shown below as a list of possible web links:
In this particular case, I chose to use the approximate values given in the descriptions of the first three links shown above. Specifically, for the length of the wall, I used the value of 13,170 miles in length. Wow! I did not realize that the Great Wall of China was that long. Of course, I have never visited the Great Wall of China, therefore, I had no real guess. Parts of the winding wall are shown below which span a large length:
Source: Wikipedia
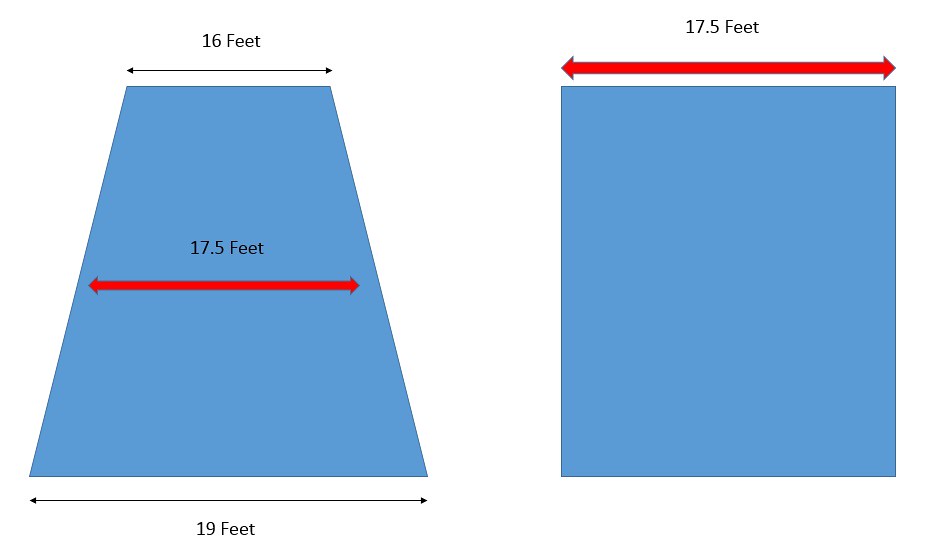
There are two remaining measurements that are needed to calculate the volume of the Great Wall of China -- the 'Width' and 'Height' of the wall. In the description contained in the image, the base of the wall is around 19.6 feet in width, whereas, at the top, the width is around 16 feet. There seems to be a large amount of variance. Given the size and the age of the structure, that is to be expected - possibly. In the image below, I show a 'trapezoid' with the values given above of the varying width if the wall with height:
With any dimensional analysis problem, the important point is to approximate the parameters (unless you have an exact value) and calculate. The problem can be turned into an 'optimization' problem where we re-evaluate the value of the parameters and then try to be more accurate in approximating the correct values of the dimensions and recalculate. For now, an initial value is important to try to find an initial answer. As I mentioned above, the length of the wall is stated to be around 13,170 miles. Therefore, below I show the values of the Great Wall of China which will be used to calculate a volume:
Note: first that there are different 'units' of measurement for different parameters. For the length of the wall, I express the value in 'units' of 'miles' instead of units of 'feet.' For the purpose of calculating the volume, the preferred unit will be the 'yard'. In order to convert from units of 'miles' to units of 'yards' the conversion factor needs to be known.
Again, asking a search engine such as 'Google' can yield an answer. If you type in the following question: How many yards in a mile? The following answer (conversion factor) appears:
The conversion factor from units of 'mile' to 'yard' is therefore used to convert the length of the Great Wall of China from 13,170 miles to the equivalent number of yards as shown below:
Converting the length of 13,170 miles into 23,180,000 yards is required to ensure that when the volume is calculated below, the final units will be expressed in 'cubic yards'. The only way to accomplish the conversion is to ensure that all three dimensions are in equivalent units of 'yard'.
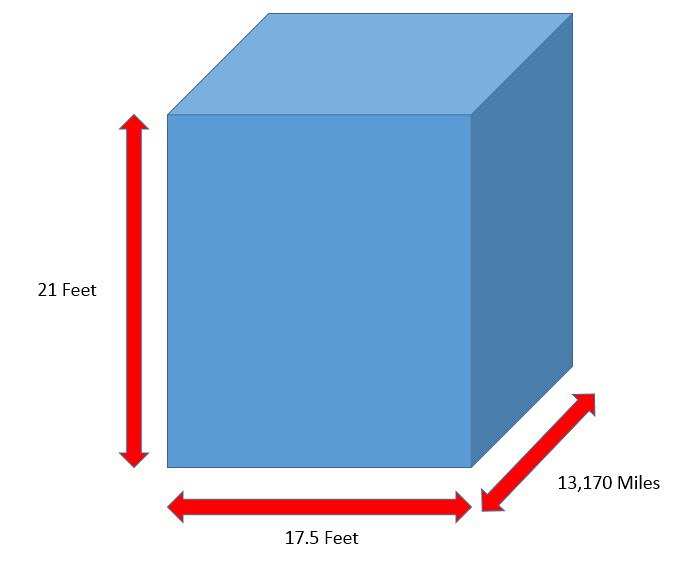
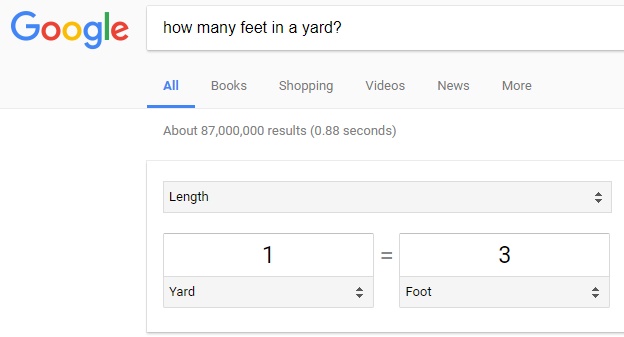
In the cartoon image of the wall above, the width and height of the wall are expressed in units of 'feet' while the length is expressed in units of 'mile'. The unit conversion from 'mile' to 'yard' has been accomplished above. The remaining unit conversions needed are of width and height. In order to do so, the conversion factor from 'feet' to 'yard' needs to be known. Next, we follow the method above of asking a search engine such as 'Google' the following question: How many feet in a yard? The answer is shown below:
Now that the conversion factor from units of 'feet' to 'yard' is known, the following unit conversions of both the 'width' and 'height' of the Great Wall of China can be accomplished as follows:
... and the height of the wall is shown below:
All three dimensions of the volume of a rectangle have been converted to the 'common' unit of 'yard'. Next, an expression for the volume of a rectangle needs to be obtained. Shown below is an expression for the volume of a rectangle:
The volume of a rectangle is defined by the area of the face of a rectangle multiplied by the length extended back into space. I define this based on the cartoon image above showing all three parameters. The length of the Great Wall of China is known as are both the width and height. To start to find the volume, first the calculation of the area of the rectangle (wall -- represented as a 'cross-section'). An expression for the area of a cross-section (or rectangle) of the wall is shown below:
From the converted values into units of 'yards' above, the following width and height can be easily plugged into the expression for the area of a cross-section of the wall as shown below:
The area of a cross-section of the Great Wall of China is 40.8 square yards as shown in the calculation above. In order to calculate the volume of the Great Wall of China, the area is multiplied by the length of the wall as shown in the expression below:
The total volume contained in 13,170 miles of the Great Wall of China is 946,000,000 cubic yards! That seems to be an enormous (and incomprehensible) volume. The above calculations satisfy the second question initially asked in the blog post. Specifically, what is the volume of the Great Wall of China. In the current blog post, the Great Wall of China is used as a metric to compare the enormous value of trash (in weight) of 150 million tons -- which was dumped over decades onto Staten Island.
How do these two numbers compare in magnitude?
That is difficult to say in the current form. Why? Because, the value of the volume of the Great Wall of China is expressed in units of 'cubic yards' while the enormous amount of trash is expressed in units of tons. A weight cannot be compared directly to a volume. Although, through the relation of a compounds 'density' - the amount of weight in a given volume -- the volume of trash may be determined as you will see in the next section.
Volume Of A 150 Million Tons Of Trash
The amount of trash which has accumulated onto Staten Island over the last few decades is around 150 million tons. According to the author of the excerpt, that amount is enough to fill the Great Wall of China. After showing the excerpt, I asked three questions regarding this enormous (and incomprehensible) statistic. The third of which is stated at follows:
3) How does the volume of the Great Wall of China compare to the stated value of 150 million tons?
As I just mentioned at the end of the last section, the route toward comparing the volume of the Great Wall of China is through comparing two enormous volumes. The first volume is that of the Great Wall of China, which was calculated to be 946,000,000 cubic yards. In order to compare this huge volume to the weight of 150 million tons of trash, a conversion from a weight to a volume needs to be accomplished.
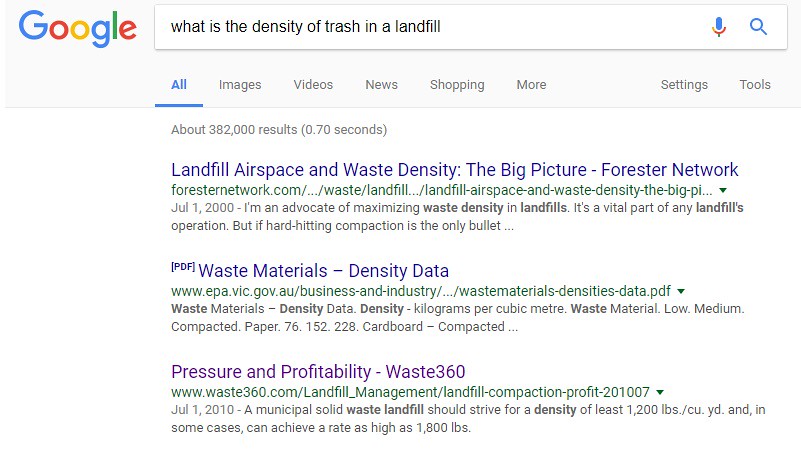
An object (in this case a pile of trash) has a certain composition of material. The material has a weight and takes up a certain amount of space. The density of such a material is defined as the weight of a material divided by its volume (which is taken up by a material). In the case of trash, a search engine can be asked the following question:
The average density of trash is around 1,400 lbs/cubic yard. Now that the density of trash in a landfill is known, the next step is to check the 'units'. Notice how the density of trash is expressed in units of 'lbs/cubic yard". That is pounds per cubic yards. Which means in order to use the value of density in the conversion, the weight of the trash must be converted to units of 'lbs' from 'ton'. To start, the weight of the trash pile is expressed in scientific notation as shown below:
The average density of trash is around 1,400 lbs/cubic yard. Now that the density of trash in a landfill is known, the next step is to check the 'units'. Notice how the density of trash is expressed in units of 'lbs/cubic yard". That is pounds per cubic yards. Which means in order to use the value of density in the conversion, the weight of the trash must be converted to units of 'lbs' from 'ton'. To start, the weight of the trash pile is expressed in scientific notation as shown below:
In order to convert the weight of 150 million tons of trash into units of pounds, the conversion factor must be obtained. If 'Google' is searched, the following answer appears below:
For every ton of trash, there are 2000 pounds. The conversion between units is easily accomplished as shown below:
The answer indicates that a 150 million tons of trash is equivalent to 300 billion pounds. Next, an expression for the relationship of mass to volume of matter must be obtained through the concept of 'density' -- which is shown below:
Since the density is known of the trash in a pile (or landfill), the expression can be rearranged to yield the volume of 300 billion pounds of trash. The expression is rearranged to solve for volume by dividing mass by density. This expression works for all matter which occupies a given space.
Plugging in 300 billion pounds for 'mass' and '1,400 pound/cubic yard' for density yields the following expression for the volume of trash as shown below:
The answer can be interpreted as the trash on Staten Island will take up a volume of 214,000,000 cubic yards. That is 214 million cubic yards of space given the compaction density of 1,400 pounds/cubic yard. Now that the volume of trash is known, the amount of the Great Wall of China (which can be filled up with trash) can be determined. This is accomplished by dividing the volume of trash by the volume of the wall as shown below:
Wow. That was unexpected. According to the calculation, only 214 million cubic yards of trash will only fill the Great Wall of China 22.6%. That is just under a quarter of the entire space in the Great Wall of China.
Conclusion
The initial statistic from the author of the excerpt given at the beginning of the blog post was the following:
By 1991, the landfill contained 150 million tons of tightly packed garbage in more volume than could fill the Great Wall of China. Fresh Kills was wetland no more.
After reading this statement, you would be led to believe that the volume of trash that accumulated on Staten Island was greater than 946 million cubic yards -- the volume of space inside the Great Wall of China. Although, the calculated percent volume which would be filled with 150 million tons of trash was only 22.6% of the volume of the Great Wall of China. Which leads to the following question:
Why is there such a difference in results? How did the author arrive at the statement? What parameters did she use in the calculation?
The questions above arise from the clarity (or transparency) of the stated statistic. This happens way too often in the media. Transparency is the best bet. I will e-mail the author and ask her how she arrived at the following result which led to the statement in the excerpt above. The exercise above shows the ease with which a person without a science background can 'fact check' or 'statistic check' a written statement in the media. Go on and try to find a 'metric' (such as the Mercedez Benz Superdome) to compare the enormous value of trash in the excerpt above. Leave your result in the comments section below. Until next time, have a great day!
Related blogs can be found here:
Dimensional Analysis Of Statistics And Large Numbers - Index Of Blog Posts
What is dimensional analysis?
How many trash carts can be filled with 80 billion pounds of trash?
How many people would be killed if 1,485 pounds of Fentanyl were distributed onto the streets in the U.S.?
What Is Dimensional Analysis?
Was The Recent Oil Spill in China The Largest In History?
LimeBike Dockless Bikeshare Riders Travel A Distance Of 13,000 Miles In Just Over 3 Weeks?
How Many Cigarettes Can You Roll With 18,000 Pounds Of Marijuana?
How Many Turkey's Are Served On Thanksgiving Day? How Many People Served?
How Much Trash Would Be Required To Fill The Great Wall Of China?
How Many Birds Per Minute Can Be Processed On A Single Line At A Poultry Processing Plant?
Hurricane Harvey Drops Enough Rain On Houston To Fill 560 Dallas Cowboy Stadiums
If Technology Fails, Use Basic Math Skills - Count Manually!!
How Much Water Is Contained In All Oceans Around The Globe?





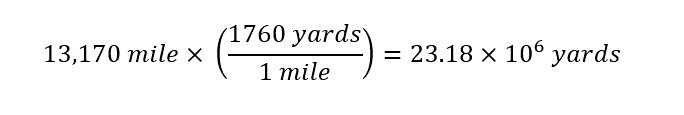
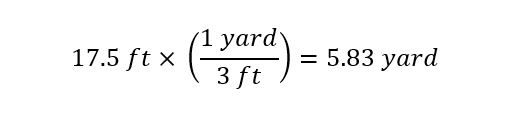
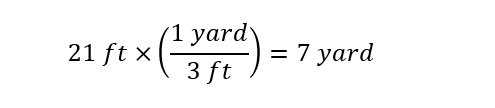
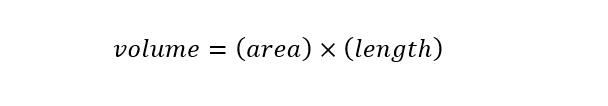
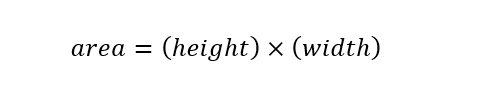
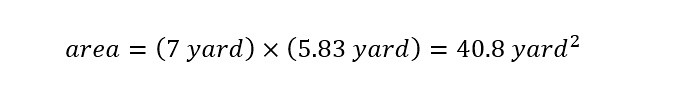
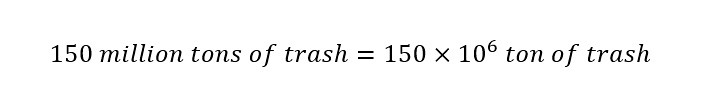
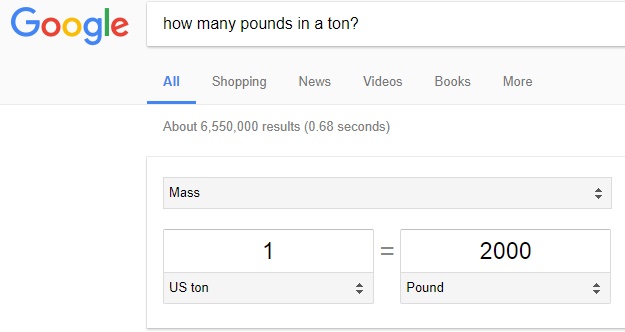
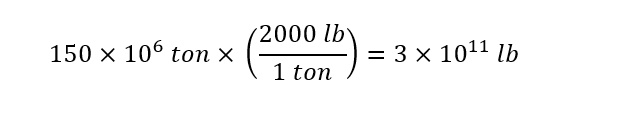
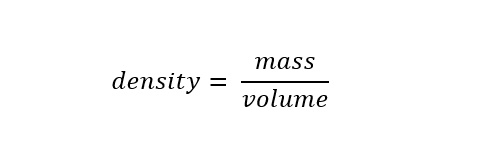
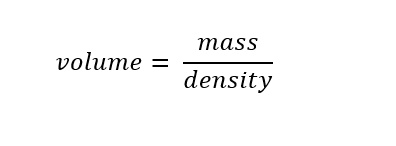
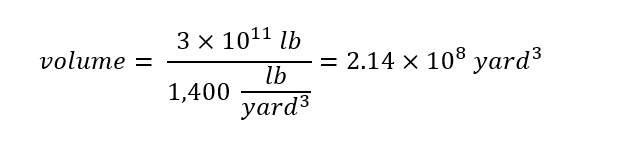
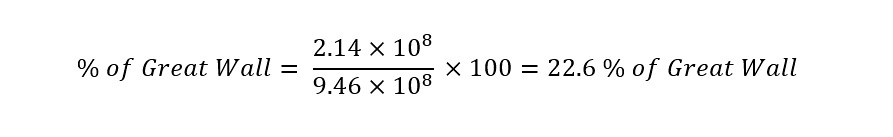
No comments:
Post a Comment